The CLIF BAR Energy Food Protein Bar For Survival Kit
The CLIF BAR looks to be one of the best energy food bars to consider, and here’s why…

The CLIF BAR looks to be one of the best energy food bars to consider, and here’s why…

An EMP can damage or destroy the electronics underpinnings within a wide variety of devices and systems that we completely depend upon.
Good radio communication etiquette and procedures for 2-way radio protocol, to help the beginner.

This is perfect if you’re looking for one of the best lightweight 12V batteries for applications such as an electric trolling motor.

Today’s popular best choices for a reasonably priced biometric handgun safe. And, a nice slider model, great for nightstand or bedside…

My favorite solar motion outdoor security light that has been running flawlessly for years and still going strong.
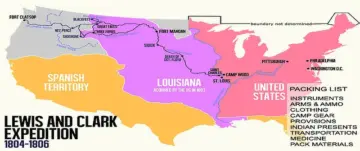
The original Lewis and Clark packing list for their Expedition of 1804 up the Missouri River.

Don’t let a perceived sense of security fool you. Gangs and Mobs will likely rule the cities after societal collapse.

Tornado season doesn’t span an exact time frame. It occurs at different times for different regions of the United States.

Paracord. The uses of paracord are nearly endless. Here’s some info about 550 Paracord, and where I get mine (made in the USA)